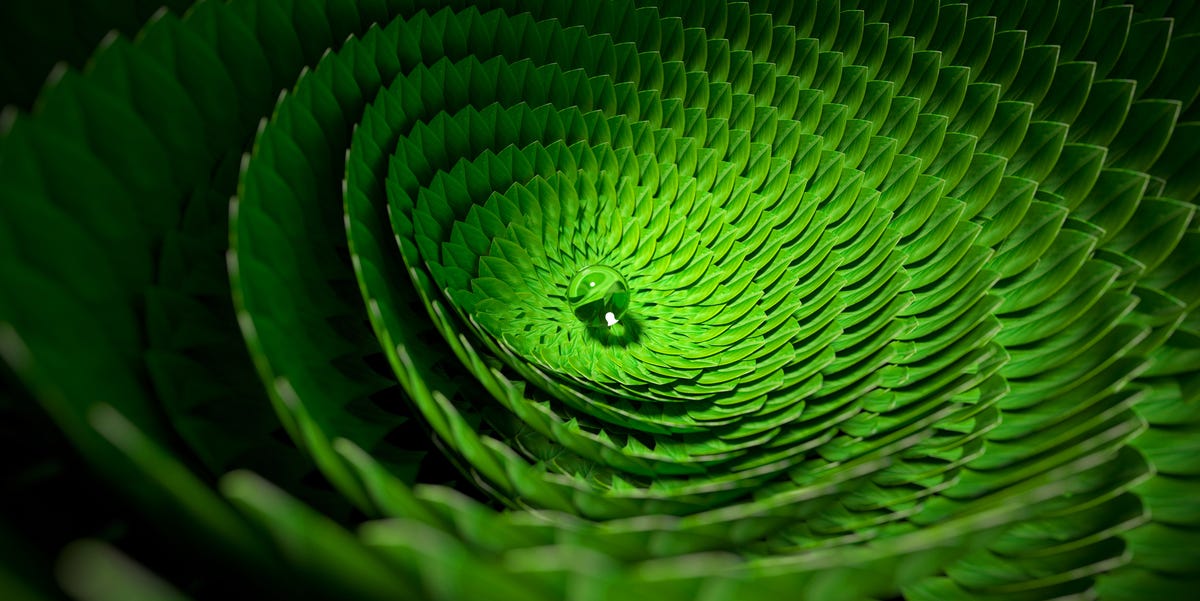
Mathematicians from Budapest University of Technology have discovered a new class of natural shapes known as “soft cells” or “z-cells,” which challenge traditional geometric tiling with their curved edges and lack of sharp corners. These shapes can tile space in both two and three dimensions, smoothly transitioning into soft tiles. This breakthrough connects the rigid world of mathematical tiling, typically characterized by shapes like triangles and squares, with the more fluid and corner-less patterns found in nature.
The research, published on the preprint server arXiv, illustrates how these soft cell shapes are prevalent in biological structures, such as muscle tissue, and are intuitively used by architects seeking to avoid sharp corners. The study also highlights seashells as a prime example of three-dimensional soft cell shapes, which exhibit a corner-less growth pattern, as revealed by CT scans. This discovery not only expands the understanding of geometric tiling but also underscores the complexity of natural forms and their departure from classical geometry.
Read more at Popular Mechanics…